1. Introduction
Cancer, as a common and frequently occurring disease that poses a great threat to human health and life, is still the main cause of death worldwide, even though its diagnosis and treatment levels are constantly improving [1,2,3]. Investigating the effect of noise on tumor growth dynamics [4,5,6,7,8,9], especially the state transition behaviors [10,11,12,13,14], via dynamic methods has become a hot topic in many cancer-related studies.
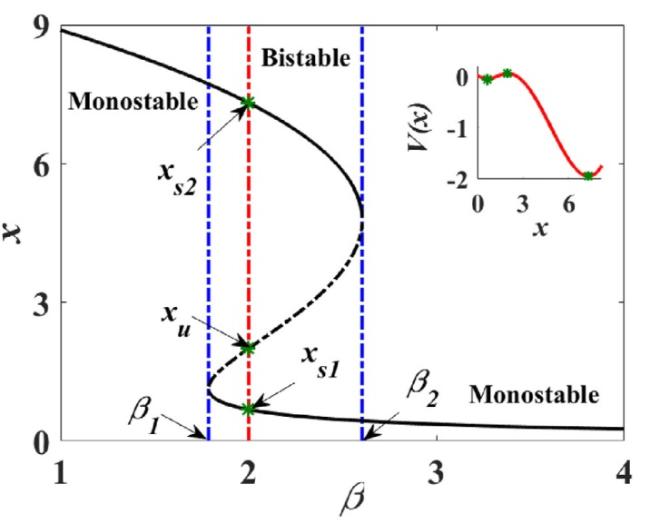
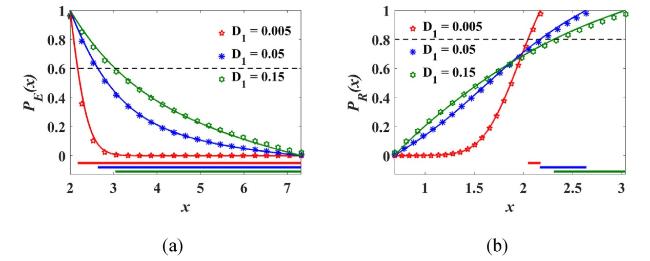
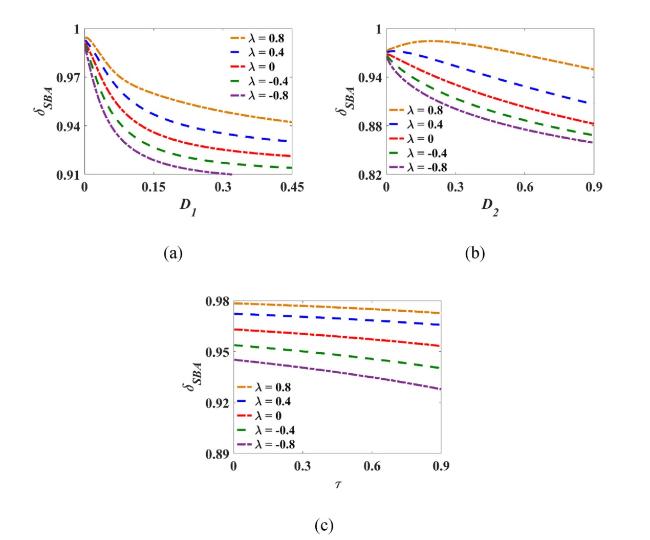
Noise-induced transition [15,16] usually refers to the qualitative changes in the state behaviors of the system induced by noise, which can be characterized by the change in the extrema of the SPDF (i.e., the emergence of new extremes or the disappearance of old extremes). Gaussian white noise has been widely applied for a long time for its advantage in facilitating mathematical processing and simplifying models. Gaussian white noise on its own fails to induce any effect on the extrema of SPDF when it is applied as an additive excitation. Moreover, the actual processes usually possess finite or even long correlation time, the $\delta$-correlation of the Gaussian white noise as an idealization of the correlation of the actual processes fails to provide a good description of the real perturbations in nature. The colored noise with exponential correlation gives a more realistic description of the noise perturbation, and in the limit case where the correlation time tends to zero, the colored noise can degenerate into white noise [17,18,19,20,21,22]. With the deepening of research and experimental verification, it is found that the noise environment in practical applications is extremely complex and often presents non-Gaussian characteristics [23,24,25,26,27,28]. When the real perturbation deviates from the Gaussian distribution, the Gaussian-type assumptions appear to be out of practice. Numerous studies related to non-Gaussian noise have demonstrated that the presence of non-Gaussian noise can induce the system to behave in richer dynamics [11,29,30,31,32]. Therefore, the study of tumor state transition under non-Gaussian noise has increasingly become a research hotspot. However, to my knowledge, few studies have focused on whether the presence of non-Gaussian noise will change the situation that independent additive Gaussian white noise can barely cause qualitative changes in the extrema. Hence, we carry out the analysis from this perspective and demonstrate that the non-Gaussian colored noise has indeed a substantial effect on the inability of Gaussian white noise on MPSS and thus contributes to the occurrence of transition induced by Gaussian white noise.
Duan et al. proposed three indexes, the most probable trajectory, first escape probability (FEP), and mean first exit time (MFET), to characterize the state transition behavior of dynamic systems [33,34,35,36,37]. Han et al. applied the most probable trajectory to the related analysis of state transitions induced by Gaussian white noise and non-Gaussian colored noise in the tumor immune system [38,39]. Hao et al. studied the Lévy noise-induced transition from the high-to-low concentrations in the sense of tumor evolution trajectories [40]. Noise-induced tumor state transition can be processed to the first escape event. The probability of the excited tumor state escaping to the nonexcited basin and the time to stay in the excited basin before the first escape occurs can be characterized by FEP and MFET, respectively. Tumor states with higher FEP or shorter MFET exhibit weaker stability and are more likely to undergo state escape. In our previous studies, tumor state transitions under the joint action of non-Gaussian colored noise and Gaussian colored noise as well as time delay were studied based on FEP and MFET, respectively [10,11]. Here, we first analyze the influence of Gaussian white noise and non-Gaussian colored noise on tumor state transitions through MPSS [30], and further emphasize the outstanding capability of non-Gaussian colored noise in inducing transitions from the FEP-dependent SBA [41] perspective and suggest the best strategy to facilitate the excited tumor state escaping to the unexcited state domain.
This paper is organized as follows. In Sect.2, the non-Gaussian colored noise is approximated and then the Markov system is derived by the UCNA method. The impacts of Gaussian white noise and non-Gaussian colored noise on the transition between the excited and nonexcited basins are discussed in terms of the MPSS and the FEP-dependent SBA in Sects.3 and 4, respectively. Sect. 5 gives a brief conclusion.
2. Model statement
$\delta\begin{array}{l} \text { Normal Cells } \xrightarrow{\gamma} X, \\ X \xrightarrow{\iota} 2 X, \\ X+E_{0} \xrightarrow{k_{1}} E \xrightarrow{k_{2}} E_{0}+P, \\ P \xrightarrow{k_{3}}, \end{array}$
where $X$represents tumor cells, $E_{0}$ stands for immune cells, $E$ denotes the mixture of $X$ and $E_{0}$, and $P$ represents the dead tumor cells. $\gamma $，$\iota$ ，$k_{i}, i=1,2,3$ and are the rate coefficients. Model (1) revealing that tumor cells transformed from normal cells can undergo reproduction, decline, and eventually die can be simplified equivalently as an univariate dynamical differential equation:
$\frac{d x_{\mathrm{N}}}{d \tilde{t}}=r_{\mathrm{N}} x_{\mathrm{N}}\left(1-\frac{x_{\mathrm{N}}}{K_{\mathrm{N}}}\right)-\varphi\left(x_{\mathrm{N}}\right) $
where $x_{N}$ denotes the tumor cell populations, $r_{N}$ represents the linear per capita birth rate, $k_{N}$ depicts the environmental carrying capacity, and $\varphi\left(x_{\mathrm{N}}\right)$ is defined as $\varphi\left(x_{\mathrm{N}}\right)=\beta_{\mathrm{N}} x_{\mathrm{N}}^{2} /\left(A^{2}+x_{\mathrm{N}}^{2}\right)$ to quantify the capacity of immune cells to recognize and attack tumor cells, two positive constants $\beta_{\mathrm{N}}$ and $A$ are used to signify the immune coefficient and the threshold that measure whether the immune system is " switched on", respectively. The dimensions of $K_{N}$ and $A$ are the same as $x_{N}$, i.e., $L^{-3}$, and the dimensions of $r_{N}$ and $\beta_{\mathrm{N}}$ are $T^{-1}$ and $L^{3}T^{-1}$, respectively. Introducing the non-dimensional quantities through $x=\frac{x_{\mathrm{N}}}{A}$, $K=\frac{K_{\mathrm{N}}}{A}$, $r=\frac{A r_{\mathrm{N}}}{\beta_{\mathrm{N}}} \beta$, $t=\frac{\tilde{t} \beta_{\mathrm{N}}}{A} \frac{1}{\beta}$, Eq. (2) can be reduced to the following non-dimensional form:
$\dot{x}=r x\left(1-\frac{x}{K}\right)-\frac{\beta x^{2}}{1+x^{2}}$
and a detailed explanation of parameters is given in Appendix A.
The potential function of Eq. (3) is
$V(x)=\frac{r}{3 K} x^{3}-\frac{r}{2} x^{2}+\beta(x-\arctan (x)).$
As shown in Fig. 1, the tumor growth system enters the bistable region when $\beta_{1}<\beta<\beta_{2}$, where there exists an excited (disease) state with a high concentration as well as an unexcited (healthy) state with a low concentration, corresponding to the two potential wells of $V(x)$, respectively. In the presence of stochastic perturbations, state transitions between the excited and unexcited states are no longer infeasible. Appropriately modulating the noise perturbations caused by tumor microenvironmental factors (such as PH, immune status, chemical agents, radiation, etc) [43,44,45] to contribute to the occurrence of the excited tumor states switching to the unexcited domain, thereby turning the system state into healthy, is crucial for the clinical treatment of tumors.
Fig. 1. Bifurcation diagram of system (3) with the bifurcation parameter. |
We characterize the impacts of ubiquitous microenvironmental fluctuations on tumor growth with non-Gaussian colored noise and Gaussian white noise, thus introducing the stochastic tumor growth model
$\begin{array}{l} \dot{x}(t)=f(x)+g_{1}(x) \varepsilon(t)+g_{2}(x) \xi(t) \\ \dot{\varepsilon}(t)=-\frac{1}{\tau} \frac{d}{d \varepsilon} V_{q}(\varepsilon)+\frac{1}{\tau} \eta(t) \end{array}$
With
$\begin{array}{l} f(x)=r x\left(1-\frac{x}{K}\right)-\frac{\beta x^{2}}{1+x^{2}} \\ g_{1}(x)=-x \\ g_{2}(x)=1 \end{array}$
$V_{q}(\varepsilon)=\frac{D_{1}}{\tau(q-1)} \ln \left[1+\frac{\tau}{D_{1}}(q-1) \frac{\varepsilon^{2}}{2}\right]$
$\eta(t)$ and $\xi(t)$ are two cross-correlated Gaussian white noises with the statistic properties
$\begin{array}{l} \langle\eta(t)\rangle=\langle\xi(t)\rangle=0 \\ \left\langle\eta(t) \eta\left(t^{\prime}\right)\right\rangle=2 D_{1} \delta\left(t-t^{\prime}\right) \\ \left\langle\xi(t) \xi\left(t^{\prime}\right)\right\rangle=2 D_{2} \delta\left(t-t^{\prime}\right) \\ \left\langle\eta(t) \xi\left(t^{\prime}\right)\right\rangle=\left\langle\eta\left(t^{\prime}\right) \xi(t)\right\rangle=2 \lambda \sqrt{D_{1} D_{2}} \delta\left(t-t^{\prime}\right) \end{array}$
$D_{1}$ and $D_{2}$ are the noise intensities of $\eta(t)$ and $\xi(t)$, $\tau$ is the correlation time of, q represents the deviation degree of ε(t).
$\dot{\varepsilon}(t)=-\frac{1}{\tau_{\mathrm{eff}}} \varepsilon+\frac{1}{\tau_{\mathrm{eff}}} \eta_{1}(t)$
is a Gaussian noise with ⟨η1(t)⟩=0, ⟨η1(t)η1(t′)⟩=2Deffδ(t−t′), Deff=[2(2−q)5−3q]2D1, and τeff=2(2−q)5−3qτ denotes the effective noise correlation time of the reconstructive colored noise in Eq. (9).
$\dot{x}=\tilde{f}(x)+\tilde{g}(x) \Gamma(t),$
where
$\begin{array}{l} g(x)=\left[D_{\mathrm{eff}} g_{1}^{2}(x)+2 \lambda \sqrt{D_{\mathrm{eff}} D_{2}} g_{1}(x) g_{2}(x)+D_{2} g_{2}^{2}(x)\right]^{1 / 2} \\ f(x)=\frac{f(x)}{\nu\left(\tau_{\mathrm{eff}}, x\right)}, \tilde{g}(x)=\frac{g(x)}{\nu\left(\tau_{\mathrm{eff}}, x\right)} \\ \nu\left(\tau_{\mathrm{eff}}, x\right)=1-\tau_{\mathrm{eff}}\left[f^{\prime}(x)-\frac{g_{1}^{\prime}(x)}{g_{1}(x)} f(x)\right] \\ =1-\tau_{\mathrm{eff}} \frac{\left[-r x^{4}+(K \beta-2 r) x^{2}-K \beta-r\right] x}{K\left(1+x^{2}\right)^{2}} \end{array}$
Γ(t) is a white noise with <Γ(t)Γ(t′)>=2δ(t−t′).
Next, we will analyze noise-induced tumor state transitions from the perspective of MPSS and SBA to obtain the best strategy for inducing transition. Unless otherwise specified, we always set r=1, K=10, and q=1.1. In addition, the UCNA regime requires that ν(τeff,x)>0, i.e., τ<(5−3q)K(1+x2)22(2−q)[−rx4+(Kβ−2r)x2−Kβ−r]x, from which τ can be restricted to τ<1.8017 when 0<x<10, 0<β<3.
3. Most probable steady states (MPSS)
In this section, the maximums of SPDF (i.e., the most probable steady states) are utilized to study the influence of non-Gaussian colored noise and Gaussian white noise on tumor state transitions under immune surveillance, especially the transition from the excited domain to nonexcited domain.
The equation controlling the extremums of the SPDF corresponding to the system (10) is derived as [30].
$m(x)-\frac{1}{2} \frac{d}{d x} \sigma^{2}(x)=0$
where m(x) and σ(x) are obtained as
$\begin{array}{l} m(x)=\tilde{f}(x)+\tilde{g}(x) \tilde{g}^{\prime}(x) \\ =\frac{r x\left(1-\frac{x}{K}\right)-\frac{\beta x^{2}}{1+x^{2}}}{\nu\left(\tau_{\text {eff }}, x\right)}+\frac{D_{\text {eff }} x-\lambda \sqrt{D_{\text {eff }} D_{2}}}{\nu^{2}\left(\tau_{\text {eff }}, x\right)} \\ -\frac{D_{\text {eff }} x^{2}-2 \lambda \sqrt{D_{\text {eff }} D_{2}} x+D_{2}}{\nu^{3}\left(\tau_{\text {eff }}, x\right)} \nu^{\prime}\left(\tau_{\text {eff }}, x\right), \\ \sigma^{2}(x)=2 \tilde{g}^{2}(x) \\ =\frac{2 D_{\mathrm{eff}} x^{2}-4 \lambda \sqrt{D_{\text {eff }} D_{2}} x+2 D_{2}}{\nu^{2}\left(\tau_{\mathrm{eff}}, x\right)} .\end{array}$
We simplify the governing equation (12) as
$\begin{array}{c} \nu^{2}\left(\tau_{\text {eff }}, x\right)\left(r x\left(1-\frac{x}{K}\right)-\frac{\beta x^{2}}{1+x^{2}}\right)-\nu\left(\tau_{\text {eff }}, x\right)\left(D_{\text {eff }} x-\lambda \sqrt{D_{\text {eff }} D_{2}}\right) \\ +\nu^{\prime}\left(\tau_{\text {eff }}, x\right)\left(D_{\text {eff }} x^{2}-2 \lambda \sqrt{D_{\text {eff }} D_{2}} x+D_{2}\right)=0 \end{array}$
It is worth noting that when τ=λ=0, Eq. (14) will be reduced to the general case that subjected to the uncorrelated Gaussian white noises, where the multiplicative noise intensity D1 becomes the dominant parameter of MPSS, while the additive noise intensity D2 disappears in Eq. (14), failing to cause the change in MPSS (see Fig. 2).
Fig. 2. The MPSS xm versus β for different D1 in (a) with D2=0 and for different D2 in (b) with D1=0, τ=λ=0. Solid lines are the extremums obtained by Eq. (14), and the star markers are the MPSS obtained by MC simulations. |
Fig. 2 presents the variation of MPSS with the immunity coefficient β for different noise intensities. We take xmb (/ xms) to denote the MPSS in the high (/low) concentration region. In comparison with the deterministic case (red), the bistable interval in Fig. 2(a) rapidly shrinks and disappears as D1 increases, meaning that the system eventually becomes monostable (green). Meanwhile, for the fixed β, the MPSS, especially xmb, is significantly reduced. It indicates that the multiplicative Gaussian white noise fluctuation generated by the microenvironment can induce the excited tumor state under different immune surveillance conditions to escape to the nonexcited region. Moreover, the enhancement of noise intensity D1 weakens the critical immunosurveillance conditions required for tumor state transition, thus improving the likelihood of maintaining the system state in the nonexcited state or even the tumor-free state. The alteration of the additive noise intensity D2 in Fig. 2(b) indeed fails to exert any effect on MPSS, which is consistent with the conclusion gained from the controlling equation Eq. (14), further confirming that the uncorrelated additive Gaussian white noise alone cannot induce the occurrence of state transition.
Next, we consider the situation of τ≠0. Fig. 3 exhibits the impacts of non-Gaussian colored noise correlation time τ on MPSS. Compared to case τ=0 with D1=0.3 in Fig. 2(a), although enlarging τ leads to an increase in the MPSS xmb, it also significantly expands the bistable range (β1,β2). This indicates that increasing τ can induce the system state that is always excited to enter the bistable region in advance (as reflected by the decrease of the threshold β1) with a weaker immune surveillance intensity, which improves the possibility of staying in the nonexcited domain. Therefore, appropriately adjusting the non-Gaussian noise correlation time τ will be beneficial in reducing the required immune surveillance intensity to stay in a healthy state when combined with immunotherapy.
Fig. 3. The MPSS xm versus β for different τ with D1=0.3, D2=λ=0. Solid lines are the extremums obtained by Eq. (14), and the star markers are the MPSS obtained by MC simulations. |
In contrast to the case of τ=0 in Figs. 2(b), Fig. 4 depicts the significant impact of the uncorrelated Gaussian white noise intensity D2 on the MPSS, especially the range of the bistability region, for a large τ. The existence of nonzero τ enables the parameter D2 that had disappeared from the controlling Eq. (14) to reappear due to the non-Markovian effect it produces, signifying that the additive white noise, aided by the non-Gaussian colored noise, not only drives the occurrence of tumor state transition but also notably reduces the critical strength of immune surveillance required for transition, exerting a beneficial role for tumor treatment.
Fig. 4. The MPSS xm versus β for different D2 with τ=0.9, D1=λ=0. Solid lines are the extremums obtained by Eq. (14), and the star markers are the MPSS obtained by MC simulations. |
Fig. 5 comparatively displays the influence of the noise correlation strength λ on the MPSS for different τ. The increase in λ in Fig. 5(a) causes an overall upward shift in the curve of the MPSS with β and shortens the length of the bistability interval, increasing the critical threshold of β required for state transition, which is not conducive to driving the system to the healthy state. Fig. 5(b) presents that a larger value of τ modulates the effect of λ on the MPSS in two ways: first, the enhancement of λ makes xmb, which originally increased gradually, decrease significantly near the bistable region; second, the increase in τ significantly reduces the bifurcation threshold β1, thus expanding the bistable interval and weakening the influence of λ on transition.
Fig. 5. The MPSS xm versus β for different λ with (a) τ=0.1，(b) τ=0.9, D1=D2=0.3. Solid lines are the extremums obtained by Eq. (14), and the star markers are the MPSS obtained by MC simulations. |
The above analysis reveals that the non-Markovian effect generated by the non-Gaussian noise exerts a significant influence on the noise-induced tumor state transition, especially the transition induced by the uncorrelated additive Gaussian white noise, completely reversing the situation that it has no effects on the MPSS transition at all.
Next, we will further analyze the role of non-Gaussian colored noise and Gaussian white noise on the escaping behaviors of tumor states from the viewpoint of SBA.
4. Stochastic basin of attraction (SBA)
The FEP P(x), which describes the probability of first escaping from B (/Bc) to Bc (/B) through the boundary xu, satisfies
$m(x) \frac{d P(x)}{d x}+\frac{1}{2} \sigma^{2}(x) \frac{d^{2} P(x)}{d x^{2}}=0, x \in B\left(/ B^{\mathrm{c}}\right),$
where
$\begin{array}{l} \left.P(x)\right|_{x_{s 2}\left(/ x_{s 1}\right)}=0, \\ \left.P(x)\right|_{x_{u}}=1 . \end{array}$
Fig. 6. Schematic diagram of tumor state transition between the excited (diseased) basin B and the nonexcited (healthy) basin Bc. |
Criterion I: PE(x)<p1∗, which gives EI={x∈B∣PE(x)<p1∗}, where PE(x) is the FEP from B to Bc through xu;
Criterion II: PR(x)>p2∗, which gives EII={x∈EIc∣PR(x)>p2∗}, where PR(x) is the FEP from EIc (EIc=Bc∪(B−EI)) to EI through xc1 (PE(xc1)=p1∗), satisfying
$\begin{array}{l} m(x) \frac{d P_{\mathrm{R}}(x)}{d x}+\frac{1}{2} \sigma^{2}(x) \frac{d^{2} P_{\mathrm{R}}(x)}{d x^{2}}=0, x \in E_{\mathrm{I}}^{\mathrm{c}}, \\ \left.P_{\mathrm{R}}(x)\right|_{x_{\mathrm{cl}}}=1 \\ \left.P_{\mathrm{R}}(x)\right|_{x_{s 1}}=0 . \end{array}$
The larger SBA means a wider range of initial states that are difficult to escape the excited domain but easier to return from outside with a higher probability. Namely, the more stable the excited domain becomes, and thus the system is harder to get out of the disease state, leading to a poorer therapeutic effect, which needs to be avoided during tumor treatment. Moreover, we appropriately set p1∗=0.6 and p2∗=0.8 to quantify the SBA.
Fig. 7(a) depicts the FEP PE(x) in the escape region B under the influence of non-Gaussian noise intensity D1. The reinforcement of D1 decelerates the descent of PE with the initial concentration x, thus inducing the critical initial state xc1|PE(xc1)=p1∗ to shift rapidly toward xs2. According to Criterion I, EI is obtained as EI=(xc1,xs2), and accordingly, EIc=(xs1,xc1). The above phenomenon indicates that the enhanced D1 significantly reduces the size of EI, allowing more excited initial concentrations to escape to the nonexcited basin Bc with high probability, thus weakening the excited basin stability and facilitating the elimination of tumor cells. Fig. 7(b) shows the trend of PR(x) from EIc to EI through xc1 under the influence of D1. In contrast to the positive dependence of PE on D1 in Fig. 7(a), PR presents a non-monotonic dependence on D1 in the region EIc. According to Criterion II, the critical state xc2|PR(xc2)=p2∗ gives the left boundary of EII, thus, EII=(xc2,xc1). Combining EI and EII, we obtain the SBA as B(p1∗,p2∗)=(xc2,xs2), that is, the position of xc2 controls the size of SBA. The enhancement of D1 significantly increases xc2, thereby shrinking the SBA, indicating that the stability of the high-concentration basin is weakened as a result of reinforcing the perturbation intensity generated by the microenvironmental factors, thus promoting the system state to becoming healthy and improving the tumor therapy effects.
Fig. 7. Effects of D1 on the FEP PE(x) in (a) and PR(x) in (b) with D2=0.005, τ=0.1, λ=0. Solid lines are the results obtained by Eqs. (15), (16), (17), and the star markers are the FEP obtained by MC simulations. |
Fig. 8 describes the effects of Gaussian white noise intensity D2 on the FEP PE(x) and PR(x) in its corresponding escape region. Compared with the PE(x) under the non-Gaussian noise intensity D1 in Fig. 7(a), PE(x) in Fig. 8(a) decreases faster, and the critical concentration xc1 in Fig. 8(a) is obviously smaller than that in Fig. 7(a) (see the boundary xc1 of EI in Table 1) under the same increment of noise intensity, resulting in a slow shrinkage of EI. In addition, PR(x) in Fig. 8(b) increases more slowly with the initial concentration under the effect of D2 than that under the effect of D1 in Fig. 7(b), which makes the growth of the critical concentration xc2 in Fig. 8(b) smaller than that in Fig. 7(b) under the influence of the same noise intensity increment (see the boundary xc2 of EII in Table 1). Combining the effects of D2 on PE and PR, we finally conclude that the contribution of Gaussian white noise to narrowing the SBA, weakening the stability of the excited basin, and promoting the escape of the excited tumor state to the nonexcited domain is significantly weaker than that of non-Gaussian colored noise.
Fig. 8. Effects of D2 on the FEP PE(x) in (a) and PR(x) in (b) with D1=0.005, τ=0.1, λ=0. Solid lines are the results obtained by Eqs. (15), (16), (17), and the star markers are the FEP obtained by MC simulations. |
Table 1. Horizontal coordinate of EI=(xc1,xs2) and EII=(xc2,xc1). |
| Intensity | D1=0.005 | D1=0.05 | D1=0.15 | D2=0.005 | D2=0.05 | D2=0.15 |
|---|---|---|---|---|---|---|
| EI | (2.17,7.32) | (2.64,7.32) | (3.04,7.32) | (2.17,7.32) | (2.28,7.32) | (2.42,7.32) |
| EII | (2.05,2.17) | (2.17,2.64) | (2.31,3.04) | (2.05,2.17) | (2.06,2.28) | (2.11,2.42) |
Next, a stability index δSBA=LSBA/LB (L denotes the interval length in R1) is introduced to deeply analyze the effects of correlated non-Gaussian colored noise and Gaussian white noise on tumor state translation in terms of basin stability. A larger LSBA indicates a more stabilized excited basin, followed by a harder escape from the disease state and a worse efficacy of tumor treatment.
Fig. 9(a) exhibits the variation of δSBA with the noise intensity D1 under different noise correlation strength λ. For λ≤0, δSBA decreases with enhancing D1, and the smaller λ hastens the decline of δSBA. That is, strengthening the non-Gaussian noise intensity contributes positively to weakening the excited basin stability and facilitating the switching to the nonexcited state. Meanwhile, strengthening the negative correlation strength (i.e., the smaller the value of λ) amplifies the significant contribution of D1 in promoting transition. For the case λ>0, δSBA behaves nonlinearly with increasing D1. The emergence of a critical intensity to maximize δSBA signifies enhanced excited basin stability with the lowest likelihood of state transition. Moreover, increasing the positive correlation strength mitigates the nonmonotonic variations of δSBA, thus expanding the scope of noise intensity unfavorable to state transition and should be eschewed within the tumor elimination stage. Fig. 9(b) displays δSBA versus D2 with different λ. Compared with Fig. 9(a), δSBA varies relatively slowly with D2, reflecting a weaker sensitivity of δSBA alterations in D2 than to those in D1. Therefore, adjusting the non-Gaussian noise perturbation can achieve better efficacy under the same intervention strength. Fig. 9(c) shows the opposite trends exhibited by δSBA along with increasing τ and λ. The decline of δSBA caused by τ facilitates the escaping to the nonexcited basin. However, strengthening λ diminishes the beneficial impact of τ on weakening the excited basin stability and inducing transition. Consequently, strengthening the negatively correlated noise intensities (especially the non-Gaussian colored noise intensity) and prolonging the correlation time are the preferred treatment strategies.
Fig. 9. Stability index δSBA under the combined influence of noise parameters, where τ=0.1, (a) D2=0.05, (b) D1=0.05, (c) D1=D2=0.05. |
5. Conclusion
Tumor state transitions under the non-Gaussian colored noise and Gaussian white noise are investigated via the MPSS and SBA, respectively. We approximate the non-Gaussian colored noise to a renormalized Gaussian colored noise and then derive the Markovian approximation of the system by the UCNA. The extremal controlling equation of SPDF is derived to analyze the impacts of noise on the tumor state transition in terms of the MPSS. Subsequently, the FEP-dependent SBA is performed to characterize the stability of the excited domain, thereby reflecting the likelihood of escaping to the nonexcited state.
The results show that (i) the non-Markovian effect generated by the nonzero correlation time of the non-Gaussian colored noise enables the reappearance of the uncorrelated additive white noise parameter that had vanished from the extremal controlling equation, implying that the existence of the ‘color’ of the non-Gaussian colored noise completely alters the situation that the uncorrelated additive Gaussian white noise fails to act on the tumor state transition. (ii) The cross-correlated noises play a dual role in regulating the SBA. The increased SBA indicates the enhanced excited basin stability, which reflects the more difficulty to escape to the nonexcited state. Therefore, enhancing the negatively correlated noise intensities and augmenting the non-Gaussian noise correlation time is essential for promoting the turnaround of the system state to be healthy and achieving optimal therapeutic efficacy.
Previous studies on tumor state transitions mostly focused on the qualitative changes in the extreme values of SPDF induced by noise as well as the transition times between the excited and unexcited tumor states or tumor-free states, where Gaussian noise was usually utilized to emulate environmental perturbations [6,52,53,54]. As an additive excitation, Gaussian white noise alone is incapable of causing an effect on the extrema. In recent years, dynamical phenomena related to non-Gaussian noise have been studied extensively [55,56]. However, to my knowledge, few studies have focused on whether the existence of non-Gaussian noise will contribute substantially to changing the non-action of independent additive Gaussian white noise on the extrema of SPDF. Here we present the analysis from this perspective and show that the non-Gaussian colored noise does improve the inability of Gaussian white noise on MPSS and further highlights the superior ability of non-Gaussian colored noise to induce transitions from the SBA perspective. It is expected that this study will provide new mindsets for tumor clinical treatment.
Declaration of competing interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
Acknowledgements
This work was supported by the Zhejiang Provincial Natural Science Foundation of China (No. LR20A020001), and the National Natural Science Foundation of China (Nos. 11932017, 11402227, 11432012, and 11621062).
Appendix A.
Table 2. Description of the variables used in the paper. |
| Symbol | Comments |
|---|---|
| x | Population of tumor cells |
| r | Linear per capita birth rate of tumor cells |
| K | Carrying capacity of the microenvironment |
| β | Immune coefficient |
| ε(t) | Non-Gaussian colored noise |
| τ | Correlation time of ε(t) |
| q | Deviation of ε(t) from the Gaussian behavior |
| D1 | Noise intensity of ε(t) |
| Vq(ε) | q-based potential of ε(t) |
| ξ(t)、 η(t) | Gaussian white noise |
| D2 | Noise intensity of ξ(t) |
